[Research] Structures of two dimensional ice predicted by computer simulations
13 January 2016
Scientists at UCL, including the LCN, and Cambridge have predicted new two-dimensional ice structures on the basis of state-of-the-art computer simulations.
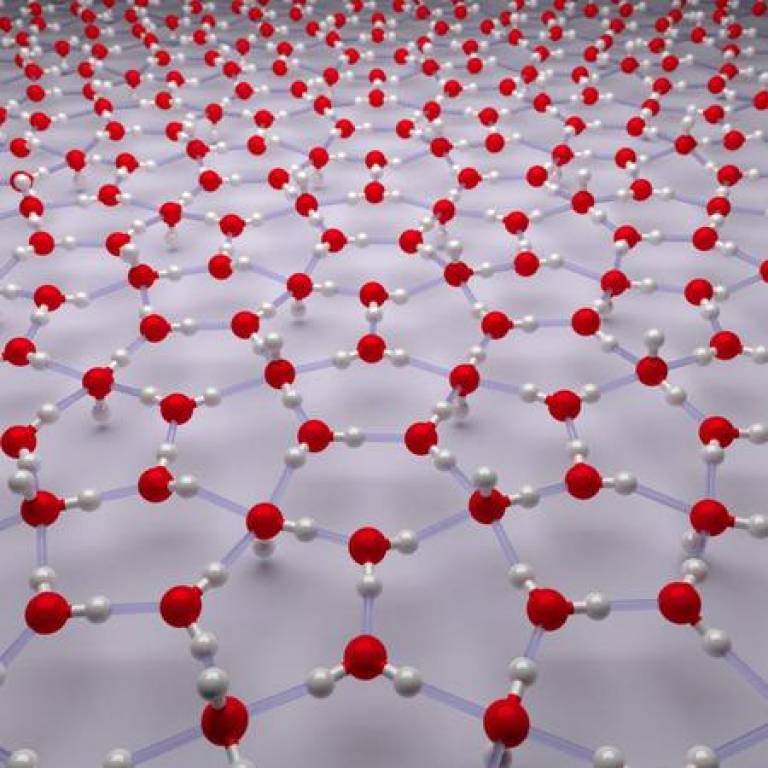
A systematic computer simulation study has led to predictions about how water molecules freeze into a single layer of ice. These simulations, published in Physical Review Letters, reveal several models for 2D ice, including a hexagonal, a Cairo tiling pentagonal, a square and a rhombic structure. The new 2D ice structures, obtained on the basis of first principles simulations and unbiased structure search methods, extend the knowledge of ice in nature and are potentially important in understanding phenomena such as cloud microphysics and tribology.
The authors also predict a sequence of phase transitions that happens as a function of pressure and confinement, leading to the determination of a phase diagram of 2D ice.
Figure: The structure of two-dimensional ice built purely from water pentagons. This space filling structure is made possible by arranging water molecules in a so-called Cairo tiling pattern.
Overall this work provides a fresh perspective on 2D confined ice, highlighting the sensitivity of the structures formed to the confining pressure and confinement width. The observation of the flat square structure supports recent experimental observations of square ice confined within graphene sheets. The authors also discuss how other structures suchas the Cairo tiling pentagonal structure may be observed by slightly altering the conditions used so far in experiments.
The publication in Phyical Review Letters can be downloaded.
Author(s):
Tag(s):
 Close
Close

